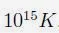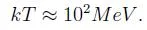Deuterium bottleneck - At high temperatures and densities, according to Hawley and Holcomb (1997) neutrons and protons can fuse directly to form deuterium (also called heavy hydrogen) nuclei, or deuterons.
Deuterium is the isotope of hydrogen, and it contains one proton and one neutron
of key fusion reactions . The  in this reaction represents a photon.This reaction liberates the binding energy (energy equal to the energy liberated when a nucleus is created from other nucleons or nuclei -Wikipedia) of the deuterium nucleus in the form of photon. Deuterium then fuses with a proton or another deuterium as in here shown in the second reaction,
in this reaction represents a photon.This reaction liberates the binding energy (energy equal to the energy liberated when a nucleus is created from other nucleons or nuclei -Wikipedia) of the deuterium nucleus in the form of photon. Deuterium then fuses with a proton or another deuterium as in here shown in the second reaction,
 in this reaction represents a photon.This reaction liberates the binding energy (energy equal to the energy liberated when a nucleus is created from other nucleons or nuclei -Wikipedia) of the deuterium nucleus in the form of photon. Deuterium then fuses with a proton or another deuterium as in here shown in the second reaction,
in this reaction represents a photon.This reaction liberates the binding energy (energy equal to the energy liberated when a nucleus is created from other nucleons or nuclei -Wikipedia) of the deuterium nucleus in the form of photon. Deuterium then fuses with a proton or another deuterium as in here shown in the second reaction,
to form the helium nucleus 3He or as shown in below 3rd reaction,
fuses with neutron to create a tritium 3H. As shown in following reaction 4
both these nuclei (3He and 3H) then react with additional particles, 3He with a neutron or a deuteron, and the tritium with a proton or a deuteron, to form 4He. This is the most common isotope in the universe and almost all helium in the universe was created in this nucleosynthesis epoch, shortly after the big bang.
We can fi nd that the equilibrium Hydrogen density is proportional to exp(B/kT) where B = 13:6eV is the binding energy of the hydrogen atom. The most strongly bound light nucleus is 4He, with binding energy B4 =28:5MeV . So most of the nucleons end up as Helium in equilibrium and that's why we should have Helium abundances now. As the universe cooled down and expanded di fferent nuclear reactions froze out, leaving the relic abundances of the stable nuclei.
Key Fusion Reactions:
Below are the Key fusion reactions of Big Bang nucleosynthesis taken from lecture
from Steven Weinberg:
4He is a very stable nuclei with close to 28MeV binding energy. However, a nuclei with atomic number A = 5 is unstable. Therefore the further fusion is rare with lower binding energies. However this would be overcome and the production of 7Li will proceeds through. These further reactions with Li production are shown here:
The weak interaction rates responsible for n - p equilibrium freeze -out at
T ~0:8MeV . The neutron to proton ratio at this is about 1/6. However when taking
into account the fact that free neutron decays prior to deuterium formation, this
ratio drops to n/p ~ 1/7. Then the 4He mass fraction is ~ 0:25.
Reference:
1. J. Hawley and K. Holcomb. Foundations of Modern Cosmology. Oxford University Press, USA, 1997. ISBN 9780195104974. URL http://books.google.co.uk/
books?id=eBFawfP8ak8C.
2.http://star-www.st-and.ac.uk/ kdh1/cos/cos17.pdf
3 http://en.wikipedia.org/wiki/Steven Weinberg